Consider the function:

\[ f(x) = \frac{x^2 - 2x + 2}{x^3} \]

Suppose you want to graph and have the equation of the tangent to the graph of \( f(x) \) at \( x = 1 \).

1. Create a new document and select Add Graphs. Enter the function.
2. Choose an appropriate window. Here, we chose:
   • \( X_{\text{min}} = 0 \)
   • \( X_{\text{max}} = 2 \)
   • \( Y_{\text{min}} = -1 \)
   • \( Y_{\text{max}} = 5 \)
3. Press , select Geometry > Points & Lines > Point on Graph. Put the point at \( x = 1 \) on the graph.

   

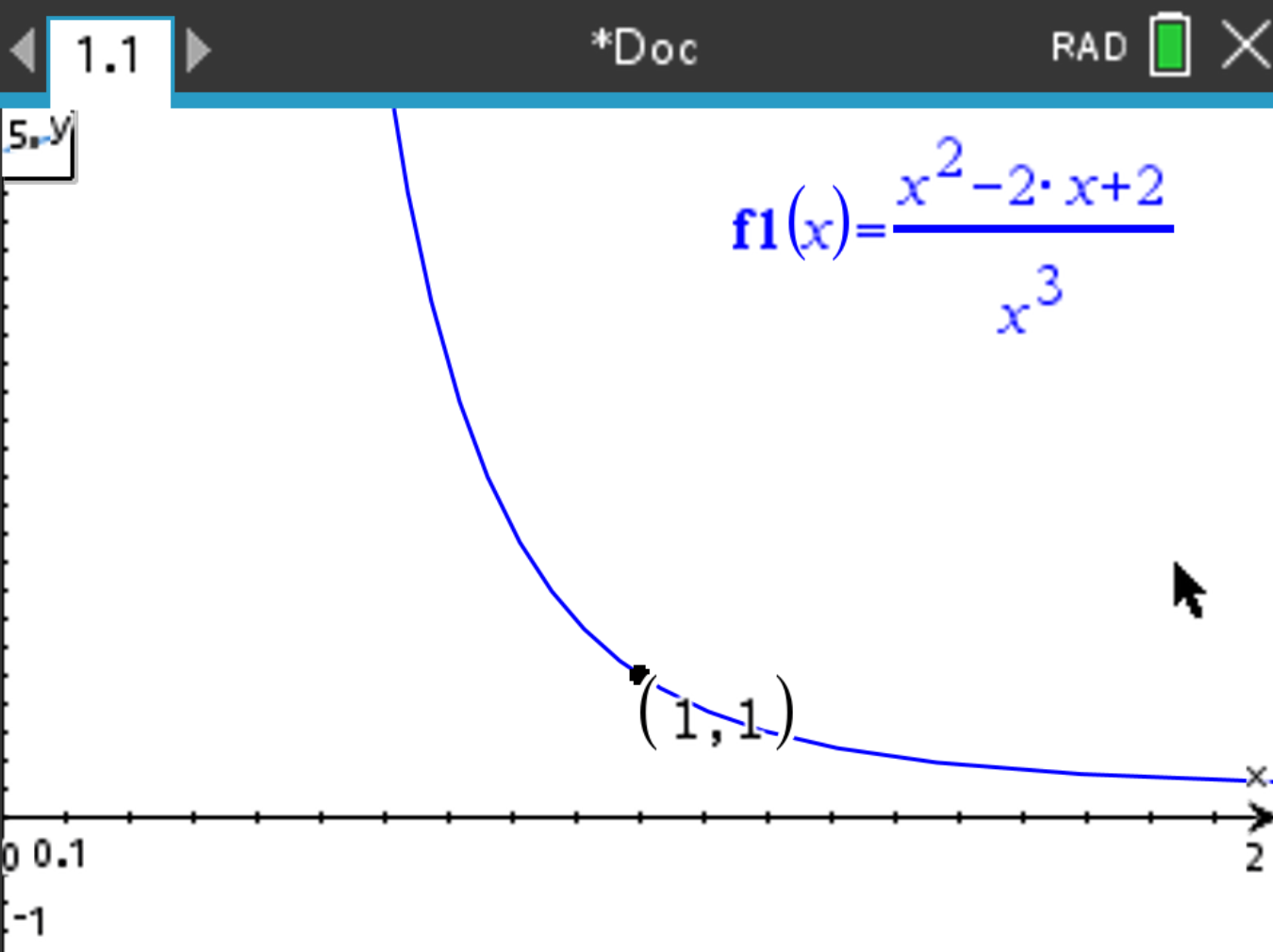
4. Then press , select Geometry > Points & Lines > Tangent and click on the point created before. The equation of the tangent is also displayed:

   

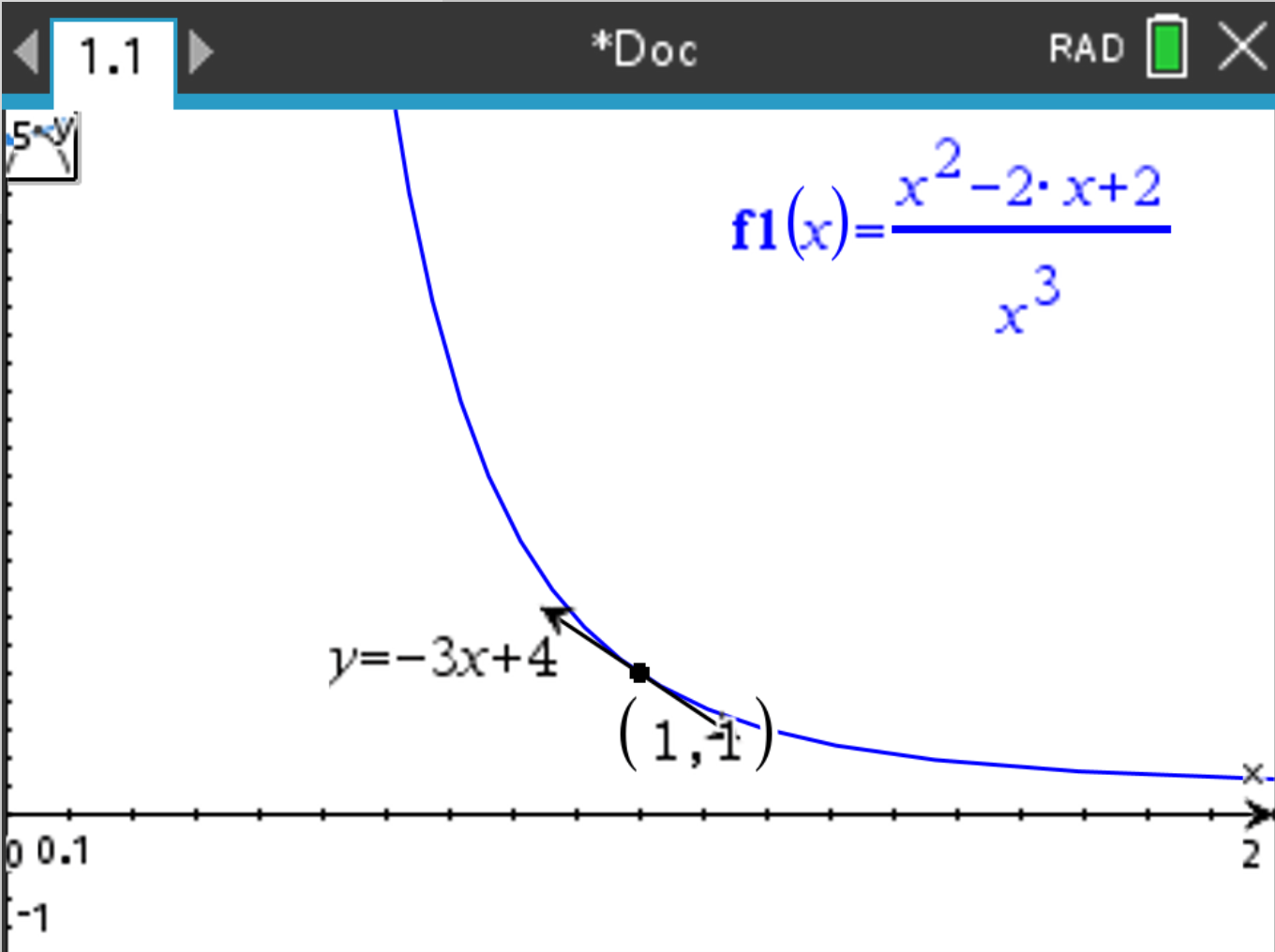
   Thus, we can read at the bottom that the equation of the tangent to the curve at \( x = 1 \) is \( y = -3x + 4 \) (rounded).